Wizard of Oz Septimal Chess
Wizard of Oz CVs are unified as concept around the idea of a complexifying Road. All the several dozen board sizes used by CV experts are feasible with differing piece-type mixes. The first having been covered are the square ones with lengths 10, 8, 9, and now 7. '7x7' warrants 3-space Yellow Brick Road, as does '8' four, '9' five, '10' six, and yet to be developed '11' seven.When a piece is located on a Road square, it moves by way of intermediate Tornado square. That effect is created by another piece or Pawn situated so that the piece moving from the YBR steps by normal move onto the other unit, not capturing or affecting the intermediary, then immediately continuing a second time by normal move in completion of the one single turn/move.
Unlike the other three WoO CVs, 8-, 9- and 10-square, on this 7x7 there is option each turn to move a Road square.
Author Frank Baum in writing fourteen Wizard of Oz books from 1900 to 1920 was influenced in adaptation by earlier Alice in Wonderland storytelling and by Native American lore, for which see 'Notes'.
Representational 'Seven' is poetically a different sort of recurring "perfect" number, than actual 'Six' (6) as mathematically the first genuine definitional perfect number, 6 being equal the sum of its divisors: 1, 2, 3. The case for Seven as embodying nobility is meant not mathematically but chiefly psychologically, that in fact worldwide across cultures, tests have shown people pick '7' as their favorite number 10% of the time, ranking first among all Arabic numerals.
In CVs topical 7x7 is utilized perhaps 15th or 18th among all board sizes. Common on 7x7 are animal/beast themes: Congo, Pink Panther, Tori Shogi all three having Gilman/Japanese/Freeling "beasts" on not insignificant 49. More numerology will be developed in follow-up WoO CVs. '13' is Devil's dozen and for like reasoning '87' is Devil's number as 100 minus 13, and each and every number should also have a name. Quasi-relevant here '7' (seven) times '13' equaling 91 is the closest 13 factor to the Devil's number.
Setup
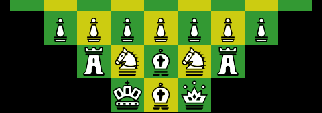
The starting array is Rook-Knight-King-Bishop-Bishop-Knight-Rook, and the WoO style makes that Tinman-Lion-Wizard-Scarecrow-Scarecros-Lion-Tinman. Seven Pawns occupy Rank Two, and Black King situates on e7, so that Kings do not face each other and opposite-color Bishops obtain.This seven by seven variant pretty well designs itself in being logical to omit Witch/Queen. Pawns have no double-step opening.
Recommended Road is e3-d4-c4, and any three-space Road allowed provides strategical intricacy notwithstanding the downsizing from 64 squares to 49 squares.
Pieces
The standard movements apply, and the specialized form of "Double Move," the Yellow Brick Road Mutator, takes effect whenever piece or Pawn stands on one of the Yellow Road squares. Typical recommended Road is that e3-d4-c4. From the initial set-up only one Black Pawn and one Black Knight can reach ybr on its first move. Again Pawn always one-steps even from array.Changes to the pieces' modes of movement occur whenever one is positioned on special Yellow square, numbering three on this board size. It is just a matter of a required Double Move from ybr square onto another piece first, then going on another movement of its type. If the intermediate Tornado cell is not available, the Piece or Pawn cannot move at all (King alone excepted).
Rules
An effect of the Pawn-no-double-step application is that Pawns cannot engage after each takes first move, unlike Octal Chess WoO.The Yellow Brick Road has the same qualification for Pawns as in the other square boards. That is, from YBR Pawn can use Tornado square of any other unit either color by its regular one-step, but by its capturing diagonal one-step, the Tornado cell must be occupied by opponent piece or Pawn other than King.
There is no capturing on the first leg off Road: Tornado square is a true pass-over cell. Likewise as in all other WoO CVs, King/Wizard has additional option to move one square along YBR without intermediary. No promotion to other than Tinman/Lion/Scarecrow.
Without a diagram yet, here is example of Knight on Road having specific moves off the Road. Say there is typical Road e3-d4-c4 more or less centralized. Knight on e3 reaches its eight squares since e3 is away from the edge. Suppose a Bishop stands on c4. Then that c4 is Tornado cell for the e3-Knight, and the actual attack squares available are the star-pattern array of Knight configuration b2-a3-a5-b6-d6-e5-e3 and d2.
Unlike the other three WoO CVs, to make 7x7 more intricate, there is option each turn to move Road space that is unoccupied; player either moves piece or pawn, or else Road cell. No moving Road square in consecutive turns. The square moved shifts location like King mode, one step straight or diagonal. There must be no occupancy of the Square moved or its arrival location. To be clear, the board stays the same, and the original square just loses its Road square character, and the arrival square then becomes YBR square. No provision is made for dis-allowing moving the ybr square to position not adjacent to the rest of the contiguous Road, but in subvariant there can be mutual agreement by the players in advance to have such enforced restriction.
Notes
How many Yellow Brick Roads are possible here? On smaller 49 cells, having the Road include sometimes squares in the first and seventh files does seem elegant and interesting, so the calculation has no such exclusion.However, there are still no (notional) 135 degree changes of direction at all in configuration of recommended Roads, as well as no 90 degree one by consecutive orthogonals in the YBRs all sizes, only either zero degrees or 45 degrees, or 90 degrees by adjacent diagonals. Violation of principle in the last sentence would just present less elegance though a game rules-set would still be reasonably operable. For this tallying purpose then, therefore, within the 21 squares a3 to a5 and g3 to g5, counting up all the possibilities of better functionality, as described above, from specific 'a4-a5-a6' to such as 'f4-f5-g6', there are 115 different Roads to choose. A lesson then is that thus even a smaller board can have challenging complexity with effective Mutator.
That author Baum draws on American Indian tradition, Legends, is not just geographical. One religious genre, that of Race with Animals, is told for thousands of years from Siberia to South America pre-colonization. Variants justify human exploitation of "beasts" despite their common origin, and there is merging of Man and Beast in all of Chess Variants, American Indian, and Oz/Wonderland.
 This 'user submitted' page is a collaboration between the posting user and the Chess Variant Pages. Registered contributors to the Chess Variant Pages have the ability to post their own works, subject to review and editing by the Chess Variant Pages Editorial Staff.
This 'user submitted' page is a collaboration between the posting user and the Chess Variant Pages. Registered contributors to the Chess Variant Pages have the ability to post their own works, subject to review and editing by the Chess Variant Pages Editorial Staff.
By George William Duke.
Web page created: 2015-11-10. Web page last updated: 2015-11-10