Not a Dodgson System Chess
Many people know the stories of Alice in Wonderland, written by Lewis Carroll. Fewer people know that Carroll was a mathematician. His real name was Charles Dodgson. In 1876, Dodgson proposed a system for selecting the winner in an election. See a scientific paper analysing this system.As people that follow modern politics will be aware of, many election systems do not guarantee that the winner of the elections is indeed also preferred by the majority of the voters. For instance, if there are three candidates, lets call them A, B, C, who are supported by respectively 46, 45, and 9 percent of the voters, but the voters in favor of C prefer B over A, then it makes quite a difference which election system one uses. In a system where the candidate with most votes wins (like in the US presidential elections (per state)), then candidate A wins; a system with two rounds, with in the second round a vote between the two candidates with most votes (unless a candidate had a majority in the first round), like in the French presidential elections makes candidate B win. But also the two-round system can give situations with an outcome, disliked by the majority of the voters, especially if there are many candidates that get a relatively small percentage of the votes in the first round. Dodgson (Lewis Carroll) proposed a system that is much `fairer', but unfortunately rather complicated. I was thinking if I could design a chess variant based on that system, but decided that that is not easy to do - players should understand the rules. So, here is a chess variant where a winner is decided, but not with a Dodgson system. Of course, the variant has elements of the famous Alice Chess, another chess variant inspired by the work of Lewis Carroll.
Rules
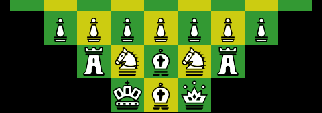
The game is played by four players. Each player plays for himself. A player has the following pieces: seven pawns, two rooks, two knights, two bishops, and one queen. The game is played on two boards, each of size 6 by 7. The setup is as follows.
Movement
Pieces move like in ortho-chess (FIDE-rules), with the following exceptions:- Pawns do not have an initital double step.
- A move must be legal on the board where it is played.
- A piece can only move or capture if the corresponding destination square on the other board is vacant.
- After moving, the piece is transfered to the corresponding square on the other board.
- When a player cannot move, he passes his turn. If he can move in a later turn, then he can and must move that turn.
Winning the game
The game ends when all pieces of a player have been eliminated. Every player gains points, as follows:- Three points for having taken the queen of the eliminated player.
- Two points for each rook, bishop, or knight taken of the eliminated player.
- One point for each pawn taken of the eliminated player.
Ties are broken in favor of the player that took the largest number of rooks from the eliminated player, and if that doesn't help, in favor of the player that took the smallest number of pawns. If there is still a tie, then there are multiple winners.
Note that only pieces taken from the first eliminated player count towards the victory.
Notes
It is allowed to make agreements. One can start by making many exchanges, or players at the same side of the board may agree not to fight to heavily at start.The principle of winning might also be tried out on a normal 2d board with multiple players.
A variant is where pawns promote to pieces that move like pawns but in all directions (mFcW), and keep a value of 1.
Written by Hans Bodlaender.
WWW page created: June 5, 2002.