Antarctic Chess or Predators and Penguins
Christmas has just passed and we have had our first major fall of snow - so something south-polar seems appropriate.
The initial inspiration came from a magazine article about a book on snowflakes and to this was added ideas arising from recent media interest in Penguins. The result is a game which offers several two-player and multi-player possibilities:
- *2-player unequal forces (2U) -Â one player controls the Predator Pack, the other controls three Penguin Colonies.
- *2-player small equal forces (2S) - each player controls a single combined group of Predators and Penguins.
- *2-player large equal forces (2L) - each player controls 3 combined groups.
- 3-player small equal forces (3S) - each player controls a single combined group.
- 3-player large equal forces (3L) - each player controls 2 combined groups.
- 4-player unequal forces (4U) - one player controls the Predator Pack, the others each control one Penguin Colony.
- *6-players equal forces (6E) - each player controls a single combined group of Predators and Penguins.
- 7-players unequal forces (7U) - one player controls the Predator Pack, the others each control one Penguin Colony.
Setup
The Board |
|
| This consists of 295 hexes
arranged in the shape of a snow
crystal. The image to the right shows this original snow
crystal†superimposed on the resulting game board. The
hexes are
shaded in one of three colours, except for the central hex which is
white. The board can also be thought of as representing pack-ice in the south-polar seas. The Penguins start at the melting, thin outer edge and their aim is to reach the safety of the centre. The Predators aim is to stop them. In game terms a player controlling a Penguin colony or a Combined group wins by moving their Emperor Penguin onto the central white hex. A player controlling a Predator pack wins by capturing all Emperor Penguins. In games with equal forces where players control one or more combined groups, a player may also win by being the only one with an Emperor penguin still on the board. |
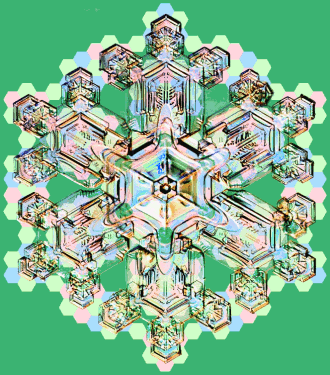 †permission
for use of the snow crystal
image kindly given by Kenneth G. Libbrecht, Professor of Physics at Caltech.
|
Pieces
| For the
Penguins we have:Â Emperor,
King,
Gentoo,
Rockhopper
and Fairy. and for the Predators: Orca, Leopard (Seal), Elephant (Seal), Petrel, and Skua. A Penguin Colony consists of: 1 Emperor, 2 Kings, 2 Gentoos, 3 Rockhoppers, and 4 Fairies. When playing against 3 Colonies the Predator Pack consists of: 1 Orca, 2 Leopards, 2 Elephants, 2 Petrels, and 6 Skuas. When playing against 6 Colonies it is doubled to: 2 Orcas, 4 Leopards, 4 Elephants, 4 Petrels, and 12 Skuas. A Combined Group consists of a Colony augmented by 1 Orca, 1 Leopard, 1Elephant, 2 Petrels, and 2 Skuas. In the file for Zillions-of-Games two piece-sets are provided. In one the pieces are represented by hexagonal tiles with a different base colour for each type of piece and a different border colour for each player. The initial letter of the piece is also shown on the tile, upper-case for Penguins, lower-case for Predators. (See images under "Initial Arrays" below right.) In the other the pieces are represented by icons in the shape of each animal. Each Piece is partially coloured to indicate the player while each Penguin also has a "waistcoat" coloured according to type. (See images under "Initial Arrays" below left.) |
|
Initial Arrays |
|
Start Position - 2U - Icon piece-set |
Start Position - 6E - Tile piece-set |
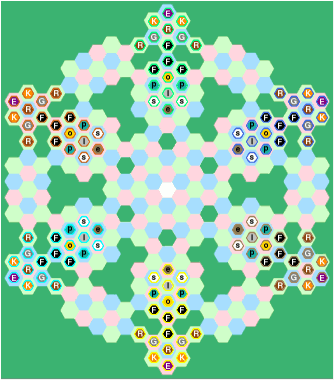 |
|
Start Position - 2S - Icon piece-set |
Start Position - 2L - Tile piece-set |
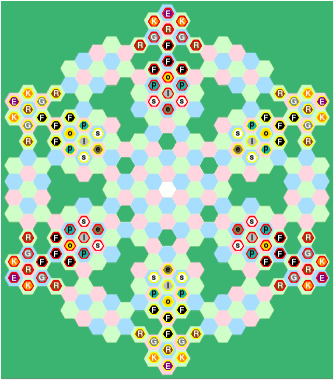 |
|
Rules
| Summary | ||||
| Variant |
Code |
Players |
Forces |
Win
Conditions |
|---|---|---|---|---|
| 2-player unequal forces | 2U |
Predators |
1 Pack |
Capture all
the Emperors |
| Penguins |
3 Colonies |
Place an
Emperor on the central
hex |
||
| 2-player small equal forces | 2S |
Gold |
1 Group |
Place an
Emperor on the central hex or capture your opponent's Emperor |
| Red |
1 Group |
|||
| 2-player large equal forces | 2L |
Gold |
3 Groups |
Place an
Emperor on the central hex or capture all 3 of your opponent's Emperors |
| Red | 3 Groups | |||
| 6-players equal forces | 6E |
Gold, Cyan, Brown, Green, Blue,
Tan |
1 Group each |
Place an Emperor or King on the central hex or be the only player with an Emperor or King on the board. |
Movement |
|
  Orca Orca |
|
| The Orca combines the move of
the orthodox Queen and Knight. It can slide in any direction any number of hexes, but may not leap over pieces that are in its path. It can also move like a knight when it may leap over pieces. A knight's move consists of a a diagonal step followed by an orthogonal step in the same general direction; alternatively the orthogonal step can be made first followed by a diagonal step in the same general direction. The Orca's moves are shown in the image to the right.  - blue dots
are reached by
sliding diagonally - blue dots
are reached by
sliding diagonally - yellow dots
are reached by
sliding orthogonally - yellow dots
are reached by
sliding orthogonally - magenta dots are
reached
by a knight's move - magenta dots are
reached
by a knight's moveA
captured Orca may be brought back into play. See the Returns section below.
|
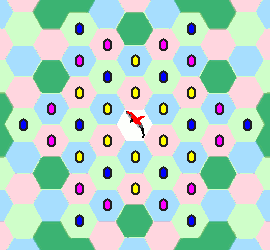 |
  Leopard Leopard |
|
| The Leopard moves like an
orthodox Queen, combining Bishop and Rook. It can slide in any direction, diagonally or orthogonally, any number of hexes, but may not leap over pieces that are in its path. The Leopard's moves are shown in the image to the right.  - blue dots
are reached by
sliding diagonally - blue dots
are reached by
sliding diagonally - yellow dots
are reached by
sliding orthogonally - yellow dots
are reached by
sliding orthogonallyA captured Leopard may be brought back into play. See the Returns section below. |
 |
  Elephant Elephant |
|
| The Elephant combines the move
of
the orthodox Rook and Knight. It can slide orthogonally any number of hexes, but may not leap over pieces that are in its path. It can also move like a knight when it may leap over pieces. The Elephant's moves are shown in the image to the right.  - yellow dots
are reached by
sliding orthogonally - yellow dots
are reached by
sliding orthogonally - magenta dots are
reached
by a knight's move - magenta dots are
reached
by a knight's moveA captured Elephant may be brought back into play. See the Returns section below. |
 |
  Petrel Petrel |
|
| The Petrel combines the move of
the orthodox Bishop and Knight. It can slide diagonally any number of hexes, but may not leap over pieces that are in its path. It can also move like a knight when it may leap over pieces. The Petrel's moves are shown in the image to the right.  - blue dots
are reached by
sliding diagonally - blue dots
are reached by
sliding diagonally - magenta dots are
reached
by a knight's move - magenta dots are
reached
by a knight's moveA captured Petrel may be brought back into play. See the Returns section below. |
 |
  Skua Skua |
|
| The Skua combines the move of
the orthodox Knight with an orthogonal step. It can step one hex orthogonally or may leap like a knight. The Skua's moves are shown in the image to the right.  - green dots
are reached by
stepping orthogonally - green dots
are reached by
stepping orthogonally - magenta dots are
reached
by a knight's move - magenta dots are
reached
by a knight's move |
 |
  Emperor Emperor |
|
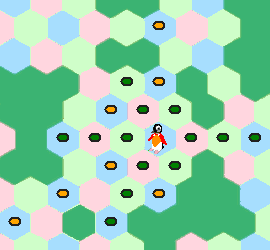
| The Emperor combines the move of
the orthodox Knight with a full double-step. It may move one or two steps diagonally or orthogonally or may leap like a knight. It may not leap over other pieces except when making a knight's move. The Emperor's moves are shown in the image to the right.  - orange dots
are reached by
stepping diagonally - orange dots
are reached by
stepping diagonally - green dots
are reached by
stepping orthogonally - green dots
are reached by
stepping orthogonally - magenta dots are
reached
by a knight's move - magenta dots are
reached
by a knight's move |
 |
  King King |
|
| The King moves with a full
triple-step. It may move one, two, or three steps diagonally or orthogonally. It may not leap over other pieces. The King's moves are shown in the image to the right.  - orange dots
are reached by
stepping diagonally - orange dots
are reached by
stepping diagonally - green dots
are reached by
stepping orthogonally - green dots
are reached by
stepping orthogonally |
 |
  Gentoo Gentoo |
|
| The Gentoo moves with a full
double-step. It may move one or two steps diagonally or orthogonally. It may not leap over other pieces. The Gentoo's moves are shown in the image to the right.  - orange dots
are reached by
stepping diagonally - orange dots
are reached by
stepping diagonally - green dots
are reached by
stepping orthogonally - green dots
are reached by
stepping orthogonally |
 |
  Rockhopper Rockhopper |
|
| The Rockhopper moves with a full
single-step. It may move one step diagonally or orthogonally to an adjacent hex. The Rockhopper's moves are shown in the image to the right.  - orange dots
are reached by
stepping diagonally - orange dots
are reached by
stepping diagonally - green dots
are reached by
stepping orthogonally - green dots
are reached by
stepping orthogonally |
 |
  Fairy Fairy |
|
| The Fairy moves with an
orthogonal single-step. It may move one step orthogonally to an adjacent hex. The Fairy's moves are shown in the image to the right.  - green dots
are reached by
stepping orthogonally - green dots
are reached by
stepping orthogonally |
 |
Returns |
|
| The Orca, Leopard, Elephant and Petrel
can all be brought back into play for their original owner by being
placed onto a hex in that player's return
area. This return area consists of those hexes occupied
by the player's Predators at the start of the game. The image on the right illustrates the return areas for variant 6E. The hexes that are in a player's return area are marked with a dot in the relevant player's colour. Returns are subject to the following rules:
|
 |
Notes
The Board |
|
| The
board was constructed by
joining 3 smaller boards. At the centre is a 5-hex per side board. This is surrounded by 6 boards with 3-hexes per side and each of these has a 2-hex per side board on each of its 3 outer corners. As well as conforming to the shape of a snow crystal the inner part of the board corresponds with the fractal curve known as a Koch Snowflake. The images below show a Koch Snowflake produced by the xfractint program, and the same Snowflake superimposed on the game board |
|
 |
 |
 This 'user submitted' page is a collaboration between the posting user and the Chess Variant Pages. Registered contributors to the Chess Variant Pages have the ability to post their own works, subject to review and editing by the Chess Variant Pages Editorial Staff.
This 'user submitted' page is a collaboration between the posting user and the Chess Variant Pages. Registered contributors to the Chess Variant Pages have the ability to post their own works, subject to review and editing by the Chess Variant Pages Editorial Staff.
By Graeme C Neatham.
Web page created: 2007-01-30. Web page last updated: 2007-01-30
